Can every conceivable map (on a sphere or a plane) be colored with four colors, or does some particular map really need five?
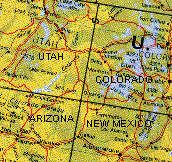
| A nice discussion of map coloring can be found in "The Mathematics of Map Coloring," which Professor H.S.M. Coxeter wrote for the Journal of Recreational Mathematics, 2:1 (1969). He began by pointing out that in almost any atlas, 5 or 6 colors are used in a map of the United States to distinguish neighboring states. | ||||
 |
"Apparently the artist did not realize that four colors would have sufficed. (It is understood that two states may be colored alike if they merely have a point in common, as in the case of Arizona and Colorado.)" This leads to the mathematical question,
Can every conceivable map (on a sphere or a plane) be colored with four colors, or does some particular map really need five? |
|||
| The question was first posed in 1852 by Francis Guthrie, a mathematics graduate student in London at the time. He had noticed the sufficiency of four colors for distinguishing the counties in a map of England. The question was passed along to several important British mathematicians (De Morgan, Hamilton), but apparently it was not seriously investigated until Cayley in 1878 challenged the members of the London Mathematical Society to solve it. From that time until its computer solution nearly 100 years later the problem stood alongside Fermat's last theorem among the great mathematical challenge of the century. Like the Fermat problem, the map-coloring question is easily stated and can easily be understood by anybody. Both problems lack any important consequences, yet have led to extraordinarily important new mathematical ideas and techniques. Both problems are alluring and elusive. | ||||
|
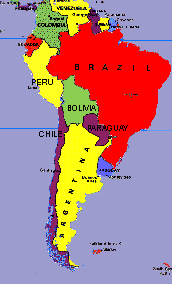
It is very easy to show that six colors suffice, and five is not much harder -- indeed, the proof that five colors suffice can be found in many elementary textbooks and expository articles. It is also easily seen that three colors are not enough -- in fact, four are needed to color Bolivia and its five neighboring States (Brazil, Peru, Chile, Argentina and Paraguay). As Francis Guthrie long ago observed, every map with only a small number of countries can easily be colored with only four colors; the challenge is to prove that four colors suffice for every conceivable map.
The proof that every planar map is four colorable was achieved in 1976 by Kenneth Appel and Wolfgang Haken, working together at the University of Illinois at Urbana-Champaign with the substantial help of a computer. An expanded version of their proof, including numerous corrections and philosophical comments, is found in their later monograph Every Planar Map is Four Colorable, Contemp. Math., vol. 98, Amer. Math. Soc., Providence, RI, 1989. Their accomplishment was announced at the University of Toronto during the 1976 summer meeting of the American Mathematical Society. The announcement set off a debate concerning the nature of mathematical proofs. |
 |
|||
|
Even though no human has every gone through Appel and Haken's entire proof, there is no doubt today that the result is correct. It has been independently checked using different techniques and different computers. For the latest word see Robin Thomas, An Update on the Four-Color Theorem, Notices of the Amer. Math. Soc. 45:7 (August 1998) 848-859 (an authoritative expository article that contains the historical and philosophical background together with an outline of the relevant mathematics and a discussion of the various proofs). On the other hand, to many mathematicians a proof must explain why the result must be true; all current proofs of the four-color theorem are a long way from that criterion.
There is an elementary text (in English) devoted to the theorem. David Barnette, Map Coloring, Polyhedra, and the Four-Color Problem. The Dolciani Mathematical Expositions,8. Mathematical Association of America, Washington, D.C. 1983. For those who read German, there is a somewhat more sophisticated, delightfully written account giving both the historical and mathematical background for the non-specialist. Rudolf Fritsch, Der Vierfarbensatz: Geschicte, topologische Grundlagen und Beweisidee. Bibliographisches Institut, Mannheim, 1994. |
||||
To return to the previous page use your browser's back button.